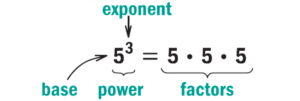
We offer free exercises on exponents and powers of numbers. The power of a number is obtained by multiplying this number by itself several times. The reader can first consult the chapter on exponents.

Mathematically, the powers of a number $a$ are defined by $a^n$ with $n$ integer. This is, $$a^n=a\times a\times\cdots\times a.$$

Selected exercises on exponents and powers of numbers

The following exercises use exponents and powers of real numbers. We assume that you are familiarised with the definitions.

Exercise: Write $1800$ as a product of powers:

Solution: Notice that $1800$ is an even number and $$1800=200\times 9= 8 \times 25\times 3^2=2^3\times 3^2\times 5^2.$$ Thus \begin{align*} 1800=2^3\times 3^2\times 5^2.\end{align*}

Exercise: Compute the number \begin{align*} \left(\frac{2}{3^2}\right)^{-3}\times \frac{2^2}{3^4}.
\end{align*}
Solution: Notice that \begin{align*}\left(\frac{2}{3^2}\right)^{-3}=\left(\frac{3^2}{2}\right)^{3}=\frac{(3^2)^3}{2^3}=\frac{3^6}{2^3}.
\end{align*}
Then \begin{align*} \left(\frac{2}{3^2}\right)^{-3}\times \frac{2^2}{3^4}=\frac{3^6}{2^3}\times \frac{2^2}{3^4}=\frac{3^2}{2}=\frac{9}{2}.
\end{align*}

Exercise: Let $a,b$ and $c$ be non zero numbers. Simplify the number\begin{align*}
A=\frac{ab^{-2}(bc^{-1})^{-2}b^3 c^2}{a^{-3}b^5 \left(\frac{1}{a^2}c^3\right)^2}.
\end{align*}Compute $A$ in the case: $a=10^3,\;b=10^2$ and $c=0.0001$.

Proof: First we calculate \begin{align*} & \left( bc^{-1}\right)^{-2}=b^{-2} (c^{-1})^{-2}=b^{-2}c^2,\cr & \left(\frac{1}{a^2}c^3\right)^2=\left( \frac{1}{a^2}\right)^2 (c^3)^2=\frac{1}{a^4}c^6=a^{-4}c^6.\end{align*} We replace these quantities in A, we find \begin{align*} A&=\frac{ab^{-2}b^{-2}c^2b^3c^2}{a^{-3}b^5a^{-4}c^6}=\frac{a b^{-1}c^4}{a^{-7}b^5c^6}\cr &=  a^8 b^{-1}c^{-2}.\end{align*} Now we take $a=10^3,$ $b=10^2$ and $c=0.0001=10^{-4}$. We then have \begin{align*} a^8= (10^3)^8=10^24,\quad b^{-1}= (10^2)^{-1}=10^{-2},\quad c^{-2}=(10^{-4})^{-2}=10^{-8}.\end{align*} We replace in $A,$ we get $A=10^{24}10^{-2}10^{-8}=10^{14}$.

Next, we give more advanced exercises on exponents and powers of numbers. Some remarkable identities are needed.

Exercise: Simplify the number \begin{align*}A=1+\frac{1}{2}+\frac{1}{4}+\cdots+\frac{1}{32}.
\end{align*}
Proof: Observe that $32=2\times 4\times 4=2^5$. Then $A$ can be rewritten as
\begin{align*}
A&=1+\frac{1}{2}+\frac{1}{2^2}+\cdots+\frac{1}{2^5}\cr &=1+\frac{1}{2}+\left(\frac{1}{2}\right)^2+\cdots+\left(\frac{1}{2}\right)^5.
\end{align*} Now we will use a remarkable identity of order 6. Then \begin{align*}
1-\left(\frac{1}{2}\right)^6=\left(1-\frac{1}{2}\right)\left(1+\frac{1}{2}+\left(\frac{1}{2}\right)^2+\cdots+\left(\frac{1}{2}\right)^5\right)=\frac{1}{2} A
\end{align*} On the other hand, we compute\begin{align*} 1-\left(\frac{1}{2}\right)^6=1-\frac{1}{64}=\frac{63}{64}.\end{align*}Thus\begin{align*}\frac{1}{2} A=\frac{63}{64}.\end{align*}
That is $A=\frac{63}{32}$.

Exercise: Let $a$ and $b$ be two distinct numbers, and $n$ a relative number. Simplify the expression
\begin{align*} B=\frac{a^n b-a^{n+1}}{ab^n-b^{n+1}}\times \left(\frac{a}{b}\right)^{-n}. \end{align*}
Proof: We factor the numerator by $a^n$ and the denominator by $b^n$ in the fraction we obtain
\begin{align*} B&=\frac{a^n( b-a)}{b^n(a-b)}\times \left(\frac{a}{b}\right)^{-n}\cr &=\left(\frac{a}{b}\right)^{-n}\frac{( b-a)}{(a-b)}\times \left(\frac{a}{b}\right)^{-n}\cr &= – \left(\frac{a}{b}\right)^{n-n}=-1 \times 1=-1.\end{align*}

We will shed some light on nth roots and rational exponents of numbers. We give a clear and rigorous definition of these root numbers. Examples and solved exercises are also given.

Generality on nth roots

We explain in a simple manner what is the nth roots of a number. We also deal with the rational exponents of numbers.

Square roots numbers and more roots

Although the square roots are standard and they are very restrictive. In fact, a number $a$ is the square root of a positive number $b$ if $a^2=b$. In this case, we write $a=\sqrt{b},$ sometimes we denote $a=b^{\frac{1}{2}}$. Thus square roots are solutions of equations of type $a^2=b$.

Naturel question: Does exists numbers $m$ positive integer number and a real number $x$ such that $x^m$ is not positive, i.e. negative? Yes, we can find examples. In fact, $(-3)^3=(-3)\times (-3)\times (-3)=-9$. Then $x=-3$ is the solution of the equation $x^3=-9$. We say that $-3$ is the cubic root of the number $-9$ and we write $-3=\sqrt[3]{-9}$. So unlike square roots, cubic roots can be negative.

More generally, let $k$ be a non-zero integer and $b$ a number, not necessarily an integer, and ask the question: Are their numbers $x$ such that $x^{2k}=b$. Nice that $x^{2k}=(x^k)^2$. Thus necessarily $b$ is positive and in this case $x$ is called $2k$-th root of $b,$ and we write $x=\sqrt[2k]{b}$ or $x=b^{\frac{1}{2k}}$. If $b$ is negative, then there is no solution. 

Thus for even numbers $n,$ of the form $n=2k$, the nth root must be positive. 

Now assume that we have an odd number $n=2k+1,$ and look for numbers $x$ satisfying the algebraic equation $x^n=b$. This is, equivalent to $x^{2k} x =b$. As $x^{2k}$ is positive, it follows that the numbers $x$ and $b$ have the same sign, either both positive or negative. In this case, the solution exists. Now if $x$ and $b$ have opposite signs, then there is no solution to the above equation.

In order to be more precise on the nth roots, let the following definition of the components of a radical expression

If we set $b=\sqrt[n]{x}$. Then we have the following cases: If the index $n$ is even: then the radicant $x$ and $b$ must be positive. If index $n$ is an odd number: then either $x$ and $b$ are positive or $x$ and $b$ are negative.

Example: take am index $n=3,$ odd number, $b=-9$, the radicand $x=-3$ is negartive.

Rational exponents

A fraction exponent, also called fractional exponent, of a number $a$ is given by $$  \sqrt[n]{a^m}=\left( \sqrt[n]{a}\right)^m=a^{\frac{m}{n}}$$ where $n$ and $m$ are relative numbers. Simply the name rational means that the exponent of the number $a$ is a fraction of the forme $\frac{m}{n}$.

A selection of exercises on nth roots

Exercise: Simplify the following expressions \begin{align*} \left(\sqrt[4]{\left(\sqrt[3]{3} \right)^2} \right)^6,\quad \sqrt[n]{3^{2n+1}},\quad \frac{5^{4}{3}}{5^{-\frac{2}{3}}}.\end{align*}

Remarkable identities are equations that simplify calculus in algebra and analysis. We give you the most famous remarkable identities, and we will provide you with beautiful proof of them.

Three famous Remarkable identities:

We recall here the most famous and used Remarkable identities.

1. The square of an addition: let $a$ and $b$ be two real numbers. Then \begin{align*} (a+b)^2=a^2+2ab+b^2.\end{align*} Preuve: Using properties of exponents, we have \begin{align*} (a+b)^2&=(a+b)(a+b)=a(a+b)+b(a+b)\cr &= a^2+ab+ba+b^2\cr&=a^2+2ab+b^2.\end{align*}
2. The square of a difference: \begin{align*}(a-b)^2=a^2-2ab+b^2.\end{align*}Preuve: We apply the above remarkable identity. In fact,\begin{align*}(a-b)^2&=(a+(-b))^2\cr &=a^2+2a(-b)+(-b)^2\cr &=a^2-2ab+b^2.\end{align*}
3. Addition multiplied by subtraction:
   \begin{align*}a^2-b^2=(a+b)(a-b).\end{align*}Preuve: \begin{align*}(a+b)(a-b)&=a(a-b)+b(a-b)\cr &= a^2-ab+ba+b^2\cr&=a^2-b^2.\end{align*}

Exercise: Find $x$ such that $x^3-x=0$.

Solution: Factor by $ x $ and using the third remarkable identity, we get

\begin{align*} x^3-x&=x(x^2-1)=x(x^2-1^2)\cr &= x(x+1)(x-1).\end{align*}Thus $x^3-x=0$ is equivalent to $x(x+1)(x-1)=0$. Hence the set of solutions is ${-1,0,1}$.

Exercise: Factor the following expressions

\begin{align*}& 9x^2-6x+1\cr &x^2-6xy+9y^2\cr &a^2x^2+2acx+c^2.\end{align*}

Solution:  The idea is to rewrite these expressions as standard remarkable Identities and then use the formula above. For the first expression, we get \begin{align*}9x^2-6x+1&=(3x)^2-2(3x)\times 1+1^2\cr&=(3x-1)^2.\end{align*} For the second expression \begin{align*}x^2-6xy+9y^2&=x^2-2x(3y)+(3y)^2\cr &=(x-3y)^2.\end{align*}For the third expression\begin{align*}a^2x^2+2acx+c^2&=(ax)^2+2(ax)c+c^2\cr&=(ax+c)^2.\end{align*}

In the case of higher degrees exponents

In this paragraph, we show remarkable identities for exponents bigger than or equal to three. These formulas are important to determine the limits of some sequences, and also to simplify expressions.

Let $a$ be a real number such that $a\neq 1$ and $n$ be a non-zero integer. We have \begin{align*}a^n-1=(a-1)(a^{n-1}+a^{n-2}+\cdots+a+1).\end{align*}  Let us give a simple proof of this identity: We set \begin{align*} A=a^{n-1}+a^{n-2}+\cdots+a+1.\end{align*}We have \begin{align*}(a-1)A&=a A-A\cr &=
(a^{n}+a^{n-1}+\cdots+a^2+a)-(a^{n-1}+a^{n-2}+\cdots+a+1)\cr &= a^n-1.\end{align*}

Let now $a$ and $b$ be two numbers such that $a\neq b$. Then
\begin{align*}a^n-b^n=(a-b)(a^{n-1}+a^{n-2}b+\cdots+ab^{n-2}+b^{n-1}).\end{align*}

Preuve: We assume that $ a $ is non-zero. Then we factor by $a^n$, we get \begin{align*}a^n-b^n=a^n\left(1-\left(\frac{b}{a}\right)^n\right)\end{align*}By using the first higher remarkable identity, we have\begin{align*}
\left(1-\left(\frac{b}{a}\right)^{n}\right)=\left(1-\frac{b}{a}\right)\left( \left(\frac{b}{a}\right)^{n-1}+\left(\frac{b}{a}\right)^{n-2}+\cdots+\frac{b}{a}+1 \right)
\end{align*}On the other hand, we write $a^n=a a^{n-1}$. We obtain \begin{align*}a^n-b^n&=a\left(1-\frac{b}{a}\right)a^{n-1}\left( \left(\frac{b}{a}\right)^{n-1}+\left(\frac{b}{a}\right)^{n-2}+\cdots+\frac{b}{a}+1 \right)\cr & =(a-b)(a^{n-1}+a^{n-2}b+\cdots+ab^{n-2}+b^{n-1}).
\end{align*}

Are you ready to unlock the secrets of exponents? These powerful mathematical tools, also known as powers or indices, are essential for mastering algebra and calculus. With a solid understanding of the rules of exponents, you’ll be able to simplify expressions, solve equations, and tackle even the most complex mathematical problems. In this article, we’ll dive into the key rules and properties of exponents, so you can take your math skills to the next level. Let’s get started!

What are exponents in math?

Have you ever heard of exponents? They’re an important concept that can help you quickly calculate large numbers. Basically, an exponent tells you how many times a number is multiplied by itself. Let’s use the number 5 as an example. If the exponent of 5 is 3, then we get another number by multiplying 5 by itself three times. That means the number 5 is repeated three times! To write this in shorthand, we use the notation 5^3. In this case, 5 is called the base, 3 is the exponent, and 5^3 is the power. Pretty neat, huh?

The present discourse aims to provide a comprehensive expression for power numbers. Consider a real number denoted by $y$ and a natural number denoted by $n$. We may express the $n$-th power of $y$ as follows: \begin{align*} y^n=\underset{n \; {\rm times}}{\underbrace{y\times y\cdots\times y}}\end{align*}

Explore the Rules of Exponents

Product of Powers

When you multiply two powers with the same base, you can simplify the expression by adding their exponents: \begin{align*} y^{n+m}=y^n y^m\end{align*}For example $$ 3^4\times 3^2=3^{4+2}=3^6.$$

Quotient of Powers

When you divide two powers with the same base, subtract the exponent of the divisor from the exponent of the dividend: $$ \frac{a^n}{a^m}=a^{n-m}.$$For example $$ \frac{5^7}{5^3}=5^{7-3}=7^4.$$

Power of a Power

To raise a power to another exponent, multiply the exponents $$(a^n)^m=a^{nm}.$$ For example \begin{align*}(2^3)^4=2^{3\times 4}=3^{12}.\end{align*}

Negative Exponents

When you have a negative exponent, move the base to the denominator and change the exponent’s sign: $$ a^{-n}=\frac{1}{a^n}.$$ For example $$ 2^{-3}=\frac{1}{2^3}=\frac{1}{8}.$$

Zero Exponent

Any nonzero base raised to the power of zero is equal to 1: $$ a^0=1.$$ In fact $a^0=a^{1-1}=\frac{a}{a}=1$.

Product to a Power

When you have a product inside parentheses raised to an exponent, distribute the exponent to each term: $$ (a\times b)^n=a^n\times b^n.$$ For example $$ (4\cdot 2)^3=4^3\cdot 2^3=64\times 8=512.$$

  Exponents worksheets

You can also consult the page on the powers of numbers where you will find some exercises with detailed solutions.

Conclusion

In the world of math, the rules of exponents are like a superhero’s trusty sidekick – always there to simplify expressions and solve equations with ease. These rules are the building blocks for more complex algebraic and calculus concepts, making them an essential tool in any math whiz’s arsenal. By mastering these rules, you’ll be able to take on any mathematical challenge that comes your way with confidence and ease. So, gear up and get ready to conquer the world of math with the power of exponents!

Get ready to dive into the fascinating world of square roots! These mathematical marvels have captivated the minds of brilliant thinkers for centuries, and for good reason. Not only are they crucial in understanding the basics of geometry, but they also have countless applications in the scientific and engineering fields.

For centuries, humans have been fascinated by the power of square roots. From ancient civilizations to modern-day mathematicians, we’ve used square roots to unlock the secrets of geometry and beyond. Take, for example, a triangle with sides of equal length – let’s say 1 centimeter each. What’s the length of the third side, you ask? Well, that’s where the magic of square roots comes in. By applying the Pythagorean relation, we can determine that the length of the third side, let’s call it $a$, is equal to the square root of 2. But here’s where things get really interesting – mathematicians have shown that this number, $\sqrt{2}$, is not a rational number. Nope, it’s a real number, and in this section, we’re going to dive deep into the fascinating world of these types of numbers. So buckle up, folks – it’s going to be a wild ride!

In this article, we’ll take a comprehensive journey through the world of square roots. We’ll start by defining what they are and exploring their properties. Then, we’ll delve into the exciting ways they’re used in both mathematics and the real world.

What is a square number?

A square number, also known as a perfect square, is a number that can be expressed as the product of another number multiplied by itself. In mathematical notation, if ‘a’ is a number, then ‘$a^2$’ represents a square number. In fact, a square is just a number $a$ with exponent $2$.

For example, 9 is a square number because it can be expressed as $3^2$, 3 multiplied by 3. Square numbers have the property that when their square root is taken, the result is a whole number.

What is a square root of positive numbers?

So, what exactly is a square root? At its core, it’s a value that, when multiplied by itself, gives you the original number. We use the symbol $\sqrt{b}$ to represent the square root of a non-negative number ‘$b$’. Sometimes we also use the notation $b^{\frac{1}{2}}$ for the square root. It’s important to note that square roots of negative numbers are considered complex numbers and are beyond the scope of this article. Are you ready to explore the world of square roots? Let’s get started!

The following rule is important:

Basic properties of square roots

Applications of this class of numbers

Are you ready to discover the power of square roots? From geometry to computer graphics, these little symbols have a big impact on a variety of fields. In geometry, the Pythagorean theorem relies on square roots to solve problems involving right-angled triangles. Engineers and physicists use square roots to calculate everything from velocity to electrical voltage. In statistics and probability, square roots help calculate standard deviations and confidence intervals. Furthermore, in finance and investment, square roots are essential for calculating risk and volatility. Even computer graphics rely on square roots to calculate distances, angles, and transformations in virtual environments. So next time you see that little symbol, remember the big impact it can have in so many different areas.

Conclusion

Did you know that square roots are more than just boring math concepts? They’re actually super important tools that have practical applications in all sorts of fields! From geometry to finance, these little symbols help us understand the world around us and solve complex problems. So next time you come across the √ symbol, don’t just brush it off as another math thing. It’s actually the key to unlocking a whole world of possibilities and real-world applications. Square roots are the foundation of math and science, and they enrich our lives in ways we might not even realize. Pretty cool, huh?

Dividing fractions may sound complex, but with the right approach, it becomes a straightforward mathematical operation. In this guide, we’ll break down the steps to divide fractions, making it accessible to everyone, from students to adults.

What is a fraction in mathematics?

Prior to delving into the division of fractions, it is imperative to possess a comprehensive understanding of the fundamental concepts. It is important to recollect that a fraction is comprised of two components, namely the numerator, which is the upper number, and the denominator, which is the lower number. The numerator signifies the proportion of the entirety under consideration, while the denominator denotes the total quantity of identical segments that constitute the entirety.

The number $x$ is called the numerator, and $y$ is called the denominator.

Maintaining a fraction in its simplified form is a more expedient approach. The simplification of a fraction renders it highly amenable for utilization in mathematical equations.

Dividing fractions: step-by-step

Are you ready to become a fraction master? Dividing fractions is a piece of cake once you’ve got the classical operators down pat. The key players here are the multiplication of two fractions and the inverse of a fraction. But wait, there’s more! You’ll also need to know how to add two functions. Don’t worry if you need a refresher on these operators, we’ve got you covered. Let’s dive in and become fraction superheroes!

The aforementioned operations on fractions provide a basis for the justification of the subsequent rule pertaining to the division of fractions.

Fractions division worksheets

Are you ready to take your fraction skills to the next level? Look no further than our exciting fractions division worksheets! These worksheets will challenge you to divide fractions like a pro, with fun and engaging exercises that will keep you on your toes. Whether you’re a math whiz or just starting out, our worksheets are the perfect way to sharpen your skills and boost your confidence. So why wait? Dive into the world of fractions division today and see just how far you can go!

Conclusion

Dividing fractions may have seemed daunting at first, but the “Invert and Multiply” rule simplifies the process significantly. With practice, you’ll become proficient in dividing fractions, a skill that finds applications in various real-world scenarios, from recipes in the kitchen to complex mathematical calculations. Remember, mastering this skill is about understanding the concept and applying the straightforward steps outlined in this guide.

The process of simplifying fractions is a fundamental mathematical concept that involves reducing a given fraction to its lowest possible terms. This process is essential in various mathematical applications, including algebra, geometry, and calculus.

How to simplify fractions

Are you ready to simplify fractions like a pro? It’s all about reducing them to their simplest form, where the top and bottom numbers have no common factors except for 1. We call these simplified fractions “lowest terms” or “reduced fractions.” So let’s get started and make math a breeze!

Simplifying fractions: Examples

Conclusion

To simplify a fraction, one must divide both the numerator and denominator by their greatest common factor (GCF). The GCF is the largest number that divides both the numerator and denominator without leaving a remainder. By dividing both the numerator and denominator by the GCF, the fraction is reduced to its simplest form. This simplification process is crucial in solving mathematical problems that involve fractions, as it allows for easier manipulation and calculation. Therefore, mastering the skill of simplifying fractions is essential for any student or individual seeking to excel in mathematics.