Are you ready to unlock the secrets of exponents? These powerful mathematical tools, also known as powers or indices, are essential for mastering algebra and calculus. With a solid understanding of the rules of exponents, you’ll be able to simplify expressions, solve equations, and tackle even the most complex mathematical problems. In this article, we’ll dive into the key rules and properties of exponents, so you can take your math skills to the next level. Let’s get started!
What are exponents in math?
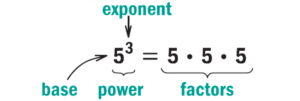
Have you ever heard of exponents? They’re an important concept that can help you quickly calculate large numbers. Basically, an exponent tells you how many times a number is multiplied by itself. Let’s use the number 5 as an example. If the exponent of 5 is 3, then we get another number by multiplying 5 by itself three times. That means the number 5 is repeated three times! To write this in shorthand, we use the notation 5^3. In this case, 5 is called the base, 3 is the exponent, and 5^3 is the power. Pretty neat, huh?
The present discourse aims to provide a comprehensive expression for power numbers. Consider a real number denoted by $y$ and a natural number denoted by $n$. We may express the $n$-th power of $y$ as follows: \begin{align*} y^n=\underset{n \; {\rm times}}{\underbrace{y\times y\cdots\times y}}\end{align*}
Explore the Rules of Exponents
Product of Powers
When you multiply two powers with the same base, you can simplify the expression by adding their exponents: \begin{align*} y^{n+m}=y^n y^m\end{align*}For example $$ 3^4\times 3^2=3^{4+2}=3^6.$$
Quotient of Powers
When you divide two powers with the same base, subtract the exponent of the divisor from the exponent of the dividend: $$ \frac{a^n}{a^m}=a^{n-m}.$$For example $$ \frac{5^7}{5^3}=5^{7-3}=7^4.$$
Power of a Power
To raise a power to another exponent, multiply the exponents $$(a^n)^m=a^{nm}.$$ For example \begin{align*}(2^3)^4=2^{3\times 4}=3^{12}.\end{align*}
Negative Exponents
When you have a negative exponent, move the base to the denominator and change the exponent’s sign: $$ a^{-n}=\frac{1}{a^n}.$$ For example $$ 2^{-3}=\frac{1}{2^3}=\frac{1}{8}.$$
Zero Exponent
Any nonzero base raised to the power of zero is equal to 1: $$ a^0=1.$$ In fact $a^0=a^{1-1}=\frac{a}{a}=1$.
Product to a Power
When you have a product inside parentheses raised to an exponent, distribute the exponent to each term: $$ (a\times b)^n=a^n\times b^n.$$ For example $$ (4\cdot 2)^3=4^3\cdot 2^3=64\times 8=512.$$
Exponents worksheets
| Exercice 1:Compare the numbers $8^4$ and $2^{12}$. | ➕ |
| We have \begin{align*}2^{12}= 2^{3\times 4}=(2^3)^4=8^4.\end{align*} | |
| Exercice 2: Find a positive number $x$ such that $x^5=2^{15}$. | ➕ |
| Let us first recall the following rule: $ a^n = b^n $ implies that $ a = b $. We will apply this property to answer the exercise. In fact, the idea is to rewrite $2^{15}$ as an exponent of $5$. Remark that $15=3\times 5$. Then\begin{align*}x^5=2^{15}=2^{3\times 5}=(2^3)^5=8^5.\end{align*}It follows that $x=8$. | |
| Exercice 3: Simplify the expression\begin{align*}A(n)=\frac{2^n+1}{1+2^{-n}}.\end{align*} | ➕ |
| We multiply $A(n)$ by $2^{-n}$, we get $$ 2^{-n}A(n)= 2^{-n} \frac{2^n+1}{1+2^{-n}}=\frac{1+2^{-n}}{1+2^{-n}}=1.$$ Thus $A(n)=2^n$. | |
You can also consult the page on the powers of numbers where you will find some exercises with detailed solutions.
Conclusion
In the world of math, the rules of exponents are like a superhero’s trusty sidekick – always there to simplify expressions and solve equations with ease. These rules are the building blocks for more complex algebraic and calculus concepts, making them an essential tool in any math whiz’s arsenal. By mastering these rules, you’ll be able to take on any mathematical challenge that comes your way with confidence and ease. So, gear up and get ready to conquer the world of math with the power of exponents!
